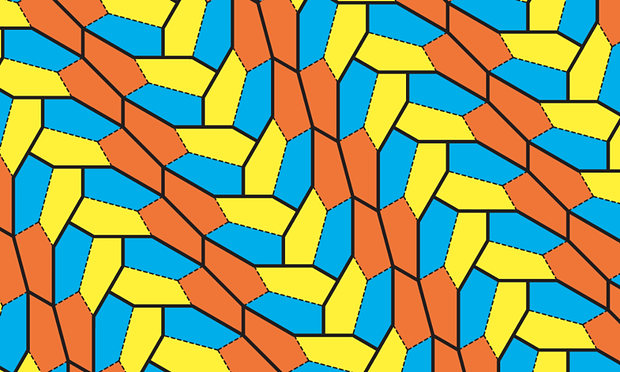
Maths/Un nouveau pavage 16082015
Un nouveau pavage pentagonal a été découvert
Découverte réalisée par ordinateur. Le précédent pavage découvert datait de 1985 (soit plus de 30 ans).
University of Washington Bothell mathematicians Casey Mann, Jennifer McLoud, and David Von Derau discovered a 15th tiling convex pentagon in 2015 using a computer algorithm (paper pending as of August 2015).
« Since 1985, while good some progress has been made on the problem of classifying convex pentagons that tile the plane, no new types had been found until 2015 with our newly identified example. Hirschhorn and Hunt proved that all equilateral convex pentagons that tile the plane are among the Types 1-14 (1985), and more recently, O. Bagina has proven that all convex pentagons that admit edge-to-edge tilings of the plane are accounted for among types 1-14. The result of Hirschhorn and Hunt and that of Bagina directed our search; from these results, we knew we needed to search for non-equilateral convex pentagons that admit only non-edge-to-edge tilings. Our new type of pentagon was identified using a computer algorithm. Using some theoretical results we have proven that limits the parameters of the search to a finite (but large) number of possibilities, we were able to develop an algorithm that can identify, for each number i, all possible convex pentagons that admit i-block transitive tilings. Our UW Bothell student, David Von Derau, worked over the course of the past year to refine and implement our algorithms to be executed on the HYAK computing cluster at the UW. We were just in the process of debugging and optimizing the code when our new example was found. Because we are in the early stages of the computational experiments, we were surprised to find this example so quickly. We are hopeful of finding more new examples as we proceed. Regards, Casey »
L'article original : https://www.reddit.com/r/math/comments/3fe347/15th_pentagon/
par Casey Mann
Actualité et pages connexes
Liens
- https://en.wikipedia.org/wiki/Pentagon_tiling
- https://www.reddit.com/r/math/comments/3fe347/15th_pentagon/
- La découverte fait l'objet d'un papier, encore non publié pour l'instant
- 10/8/2015 «Il n’y a pas de frontière entre maths pures et appliquées» https://lejournal.cnrs.fr/articles/il-ny-a-pas-de-frontiere-entre-maths-pures-et-appliquees